In recent years, poker has become very popular. Most sources from which one could learn poker strategy, such as books, instructional videos, or other digital content, have become outdated.
The fundamental change is that old-school players made millions by exploiting their opponents' mistakes and weaknesses. Nowadays, those who make piles of money are not only those who can exploit their advantage and opponents' mistakes but also those who have a good understanding of game theory. This factor elevates poker to a whole new level.
In this article, we will discuss:
- The basics of game theory
- Why you should use a game theory-based strategy
- Examples from Doug Polk, who highlighted the importance of game theory
- 4 reasons why it's worth using such a strategy
Game Theory and Poker
John Nash developed game theory as a branch of mathematics at Princeton University around 1950. Over the past 15 years, poker has become very popular, and players have reached a very high skill level. At such a level, it is very difficult to be a good player and win without the help of game theory.
Mathematical understanding has made the game much deeper and more complex. Based on game theory, you have to think through all decisions, from every opening from different positions to seemingly insignificant checks on the river when playing for a small pot. Every decision determines how much you win and lose (win rate) as a poker player. These things are measured by the value we expect or EV (expected value). If your decision is profitable, it is EV+; if not, it is EV-.
A very simple example of applying game theory is a player using a specific opening range from the UTG position.
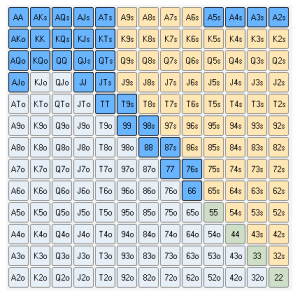
Of course, it is obvious that playing very strong hands by opening from UTG is a profitable decision, but if we only played with the strongest hands, we would be very predictable. By opening hands like 9s8s or 66, we balance our opening range and make the game more difficult for our opponents. This way, we can form a strong combination regardless of whether high, low, or medium cards are revealed on the table.
For example, the previously mentioned cards would fit:

Why Use GTO (Game Theory Optimal)?
You are probably wondering why this theory is needed if most of the money comes from exploiting the weaknesses of worse players?
Here are two main reasons:
- Using GTO, you will win money in the long run regardless of whether you are playing against weak or more skilled players
- It is much easier to adapt and change your strategy based on opponents when you have a foundation from which you can deviate depending on the opponent
If you look at the game through the GTO lens, you can review your played hands and determine what the most optimal decision would be, thus evaluating your game more objectively. This perspective allows you to understand whether you are balancing your range. Moreover, you analyze not only one specific situation where specific two cards are dealt but also generally understand how to play in such a situation with all other cards.
If in one situation you make a value bet, your range should also include hands with which you would bluff in the same spot, so your opponent can never be sure whether you are bluffing or have a strong combination. If you only bet on the river when you have a strong hand, the opponent can always profitably fold because they know you never bluff in such a situation. On the other hand, if you bluff too often in such situations, the opponent can always profitably call because they know you almost never have a strong combination.
If you still don't think a GTO-based strategy is the way to go, these hypothetical examples from Doug Polk should help.
Poker Theory, Examples
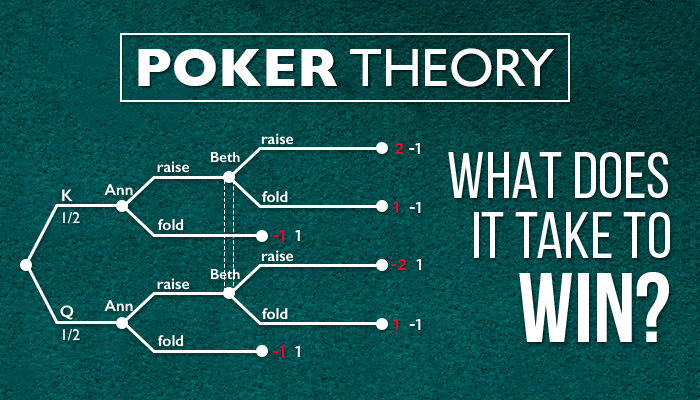
On the river, you bet $100 into a $100 pot, so your opponent needs to call $100 to win a $200 pot. This means your opponent has 2 to 1 pot odds and needs to win at least 33% of the time to break even.
This short calculation shows the optimal bluffing ratio in your range when betting on the river card. So, 33% means that one out of three times you will be bluffing, and two times you will be betting for value. This frequency is optimal because you will usually win the pot and not risk being figured out.
Let's look at 4 different scenarios of value betting and bluffing ratios to understand why a range with 33% bluffs and 67% value bets is optimal and why your opponents can't do anything against you.
To simplify the scenarios, let's imagine that we always win when the opponent calls our value bet and always lose when they call our bluff.
Scenario 1 – 0% bluff, 100% value bet
Your opponent can fold 100% of the time. In your results, this will reflect as winning $100 with your betting range.
Scenario 2 – 100% bluff, 0% value bet
Your opponent can call 100% of the time. In your results, this will reflect as losing $100 with your betting range.
Scenario 3 – 50% bluff, 50% value bet
If your opponent calls 100% of the time, you will win $200 every time you bet for value and lose $100 every time you bluff. In your results, this will reflect as winning $50 if the opponent always calls. (50% * -$100 = -$50; 50% * $200 = $100. $100 – $50 = $50).
If the opponent always folds, you will win $100 (same as in the first scenario).
This scenario shows that not bluffing at all is as profitable as bluffing 50% of the time.
Scenario 4 – 33% bluff, 67% value bet
If your opponent always calls, you win $200 when you bet for value and lose $100 every time you bluff. In this case, you lose $100 only 33% of the time and win $200 67% of the time, meaning your profit is $100. (33% * $100 = -$33; 67% * $200 = $133. $133 – $33 = $100).
This ratio of bluffs and value bets is optimal because:
- You win $100 if your opponent always calls
- You win $100 if your opponent always folds
So, you earn $100 regardless of what your opponent does. Of course, this win-win scenario is only possible when your range is perfectly balanced. Therefore, it doesn't matter what your opponent chooses, as you earn the same amount in both cases.
Applying such a balance while considering opponents' mistakes and weaknesses can be even more profitable, but it requires reliable information about the opponent and correct application. If you want to move up the limits and crush your opponents in the long run, GTO is essential.
4 Reasons Why It's Worth Using This Strategy
Let's review the benefits of GTO in our strategy, 4 things you will achieve by incorporating this theory into your game.
Avoid Illogical Thinking
The legacy of 90's poker training is trying to understand what level a player is playing at.
- The level where a player only thinks about their own hand
- Then they start considering what the opponent might have
- The next level is thinking about what the opponent thinks about your cards
- Then it starts thinking about what you think about what the opponent thinks you have
- And so on.
Ideally, you somehow figure out where the thinking ends, determine the level of the opponent, and adapt accordingly. But the reality is different, and determining the level when playing against a weak player is very unreliable. And against good and experienced players, this process can theoretically continue until the end of the world, with one outthinking the other.
Here is a great example where two poker legends are leveling each other.
https://www.youtube.com/watch?v=-hVwpaDH0Xo
I would never question Patrik Antonius's poker skills, but such situations can be avoided by basing your bluffing strategy on GTO, which helps avoid leveling wars that cause confusion and lead to situations where you bluff without equity.
Avoid Incorrect Assumptions
Another advantage is that when you look at the game through the GTO lens, you avoid making incorrect assumptions about other players. Of course, some assumptions are possible if you have more than enough hands played against a specific player, but very generalizing assumptions can be costly.
For example, it wouldn't be wise to say “there will NEVER be a bluff here” or “he will ALWAYS bluff here.” You also shouldn't think that an unknown opponent can't have a specific hand in their range or that they open very narrowly or very widely.
A well-structured GTO strategy removes confusion from your game and helps you play profitable poker in the long run.
Objective Analysis
Most players judge themselves for playing a hand poorly just because they didn't win it. However, as you improve and progress in your poker career, you begin to understand that poker is not a business where results can be evaluated in a vacuum.
Thinking objectively can be very difficult, especially when the result of a played hand is very bad or very good. Just because you caught a full house on the river and won a lot of chips from your opponent doesn't mean that calling your opponent's bet twice was a good play.
When you figure out what the most profitable decision according to GTO is in a specific situation, include the hand in your session analysis to find out if you made a long-term profitable decision not with a specific hand but with your range.
All successful poker players know that admitting your own mistakes is essential if you want to play well. GTO provides the foundation that makes it much easier to spot mistakes.
Easier Adaptation
Why is theory important when trying to adapt to other players? To make it simpler, let's play a game.
Imagine you forgot everything you knew about poker strategy except for the basic knowledge of the game, and you are playing your first hand ever.
Live game, blinds $1/$2, effective stack $200.
Hero gets Ad9d and is in the BB position. Everyone folds to the BTN, BTN raises to $7. SB folds, hero calls.
Flop (pot $14) As Td 3h
Hero checks, BTN bets $9, hero calls.
Turn (pot $32) Jc
Hero checks, BTN bets $21, hero calls.
River (pot $74) 9c
Hero checks, BTN bets $50, hero calls.
BTN shows Ah 2c. Hero wins $174 with two pairs.
What will you do next time you face this player in such a situation, how can you change your game to exploit the opponent's weakness? Without any theoretical understanding of this situation, you won't know where to start.
On the other hand, if you know how to theoretically play A2o from the BTN position, you will also know how your opponent deviated from that. This knowledge simplifies the path to adapting to this opponent.
Here are specific adjustments we can make to crush the opponent's aggressive thin value strategy.
- Small exploitation. Call the opponent's bets more widely (with weaker combinations than usual) on all streets when they bet. (don't overdo it)
- Large exploitation. Ruthlessly attack their checking range when they are obviously very weak, with large bets both with thin value and a reasonable amount of bluffs.
Very often, understanding the theory of optimal play helps to exploit opponents' weaknesses more easily because you know exactly where your opponent's decisions deviate from optimal play. When you don't know what is good, it's almost impossible to understand what is bad.
To Summarize
Striving for a perfect GTO strategy might seem like a logical conclusion, but the truth is that no one can play perfectly according to this theory. Poker will eventually be solved by humans or machines, but we still recommend basing your game on this theory as much as possible. As always, this means you have to work on your game both while playing and during off-game hours.
This article only summarizes the basic principles of game theory applied to poker, but we hope you found something useful or at least sparked your curiosity about how to enrich your game by applying game theory.